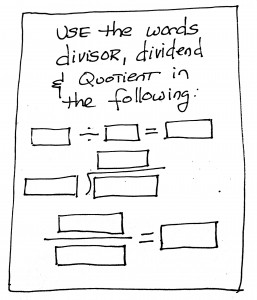
As a math coach, Robert has a very simple philosophy that he developed and now rigidly adheres to. If you are a math coach (or you work with one), you should listen up, because it will be very useful:
- The Vidal Sassoon Principle: If you were watching tv in these United States in the 70s or 80s, you’ll probably remember the commercials for Monsieur Sassoon’s hair products, and his accompanying tagline: “If you don’t look good, we don’t look good.” That same philosophy embodies Robert’s work as a math coach: his job is to make his teachers look as good as possible, which means making sure they have all the materials they need, understand what they are doing, and if anything goes wrong, taking the bullet for any mishaps. If Robert can make a teacher look brilliant, then it must be due to the fact that he is brilliant as well. In short, Robert always has your back.
This principle (one of several which I will be forced to explicated upon in future blog posts, I’ve been told) was put to the test a few weeks back when Newbie Teacher confessed that she was nervous about introducing “long division” to her class. Robert understood: everybody gets nervous teaching division of any kind (buy this and read why…), but there are ways to approach it without getting everybody nervous.
So Robert decided that maybe the best way to approach this was not “head on,” but through the “back door” (please, no salacious jokes about that….) He tossed this out to Newbie Teacher, “Okay, I know this is going to sound crazy, but let’s try this….” and he scribbled the following problem on a piece of paper:

A teacher gave this problem to her students without teaching long division. So sue her!
The teacher looked at it, said “WTF, Robert, how are they going to do this without long division?” Robert’s response? “I don’t know, but let’s find out: you’ve been teaching them all sorts of things about division for the past 3 weeks, I’ll bet they know enough to figure it out.” And so Newbie Teacher, who knew Robert’s “Vidal Sassoon” Principle, “went for it.” She put the class into small groups, showed them the problem they were to solve, handed out large sheets of paper to record their thinking processes, and only stepped in when she saw flaws in logic or explanation. She did not say anything about long division.
Here are a few of the solutions the students came up with:

You can see how the student understands that to figure out the problem, he’ll need to figure out how many times 385 goes into 14,382 (notice that Robert did not make the answer come out “evenly.” That’s because 90% of division problems don’t!) Like the standard algorithm, this student estimated and checked the answer. Unlike the standard algorithm, he didn’t get frustrated and give up: rather, he made another estimate, checked it and then skip counted until he had enough seats.

This student started with 5 planes, saw it was way too low, and then doubled it, then doubled it again, and then used the 10 plane amount and 5 plane number to get to 35 planes, and then counted on to the right number of planes. Notice that he understood that a 38th plane would be needed, even though the answer is technically 37.

When the students had all finished making their posters, Newbie Teacher showed me something cool: she had her students hang up their posters and then read one another’s methods and put “sticky notes” to either praise or question what they had done. Here’s one of the notes below:

As you can see, this is getting to the heart of the standard algorithm for long division: estimate by using the largest “guess” possible, and then add on more groups until you get as close as possible.
This activity was followed by a lesson on “multi-step” division using a “modified algorithm” that Robert prefers to teach to young children, as it is transparent, forgiving and consistent with children’s understanding of division. You can read about that in the product shown below!